One World Pragmatism is a philosopy developed by Alexander Curtis that uses the insights of science, logic and mathematics to arrive at a view of the world in which the Big Bang, the Law of Entropy and chaos theory play a central role. This is complimented and augmented by evolution, the objective nature of problems and their solutions and the thus derived, objective nature of good and bad. The philosophy is based on a three-fold form of pragmatism:

i) the accounts given by science, of the material world and how it works are accepted.

ii) following the example given by Alain de Botton in his examinations of works of literature, this impartial and unethical view of the world, is filled out by taking the complexity of life and the universe and asking whether it has anything to tell us about how we should live our lives. From chaos theory, the lesson emerges that we should respect the fragile and intricately interwoven nature of the natural world.

iii) the current state of the environment is seen as a direct result of inadequacies and oversights of modern culture. The pragmatically derived consequences of this are that the over-simplistic materialist view of the world held by many people, the overinflated myths of freedom that are part and parcel of contemporary consumer culture and the absurdly primitive idea that the only thing that counts in life is money, are all seen as false for the simple reason that they are causing us to destroy our environmental niche and with it ourselves. In ii) a re-examination of anlythic philosophy and mathematical logic dusts down a proof that given complex, self-reflective forms of life, then there is a rationale that lies over and above us and which it is our job to respect and for our own good, to venerate. This is given pragmatic support by an anthropological study it has been found that a shared set of values and beliefs is esential to the emergence of complex socities. Importantly, this Oxford University co-ordinated study has found that it is not the fear of a punishing god that is the motivating factor in bonding individuals together but rather the benefits that arise from a shared sense of identity and values. In this wider, anthropological sense, One World Pragmatism is therefore proposed as something that is essential to our survival if we are to learn how to see and interact with the world in a way that will result in a sustainable future with happy people.

Accepting that what the world needs is a shared system of belief that indicates how our survival is to be ensured, it must be observed that One World Pragmatism is a philosophy and therefore is not something that can be readily embraced by those who are not philosophically inclined. It is thus at best a skeleton which must be fleshed out in ways that give the themes and tenets expounded a presence and reality that goes beyond the cerebal. This of course, is something that must evolve as the result of a widely-based social consensus. Although not something that can be worked out by a single person or even a group of people, individual people and groups of people can contribute to the bringing into being of a cultural environment in which ideas are bounced around. From out of such a culture, there is then a chance that that which is needed may emerge. To this effect, the Reloading Humanism People Page presents people from different fields whose work is concerned with new approaches and new dialogues.

As a preview of what the philosophical skeleton has to offer, five major questions are asked, with the  answers indicating something of the approach taken.

Question 1: Do we have an immaterial self?

Yes. This however is made out of an immaterial aspect of the physical world and is not necessarily immune to death. The uncertainty here is related to the uncertainty that surrounds attempts to answer Question 5.

Question 2: Do good and bad exist?

Yes. Wherever there is life, there are for the living things concerned, problems. For any problem, there are always good and bad solutions. Thus given life, there is good and bad.

Question 3: Do we have free will?

Yes. Although everything in the world happens by cause and effect, effects are no longer seen as always being 100% predictable. This begins with the thousands of bio-molecular processes going on simultaneously in our bodies and reaches out to embrace the planets of the Solar System. Following on from these insights is the fact that what a human being is as a person and as a legal entity, is a collated construct that consists of layers and layers of systems whose interactions are so complex and replete with self-referring-loops that they are in no way deterministic.

Question 4: Is there a God in some highly abstract, expanded sense?

Yes. Given that there is good and bad and that self-conscious, living things have hopes and fears concerning the future, it has been proved that, in an abstract and expanded sense of the word, a form of god or good fortune does then exist.

Question 5: Is the God of Question 4 capable of intervening in worldly affairs?

Although modern logic and analytic philosophy have brought much clarity to a wide range of issues, they are also simply ways of seeing the world and as modern logic itself has shown, no one logical system can prove all truths that can be expressed by the system. Extrapolating out from this, means that no one way of looking at things will bring to light all that is true in the world. It may well be that in seeing the world through the mind-set of the modern materialism, it is precisely here that something has been lost. Confirming this, in what constitutes nothing less than a second Copernican Revolution, physicists now recognise that 75% of the mass of our universe is completely and utterly unaccounted for. Leaving bone-head materialism behind, both modern logic and physics do appear to be capable of providing tentative and sketchy indications of how an interventionist divine principle might operate.

Abstract

The derivation begins using the techniques of analytic philosophy before moving over to consider the status of human knowledge as a whole. Thereafter it is shown how pre-war debates on philosophy of mathematics paved the way for Alan Turing’s cracking the Enigma code used by the Germans during the war and how these debates also feed into discussions on the nature of the good and proofs of God. The thus introduced war-time theme is then continued in a consideration of the work of the Protestant theologian, Dietrich Bonhöffer, who as an active opponent of the NS regime, was arrested and executed. Early on, Bonhöffer diagnosed the nihilism into which the modern world is sliding and worked on the beginnings of a way out. Prompted by this and with recourse to mathematics, a thought experiment is conducted which enables a mutually dependant conception of the self and God to be arrived at.

The Derivation

Science tells us that the world is composed of some form of matter/energy that is arranged in space and that our universe evolved through processes of evolution with the evolution of ourselves and our ability for self-conscious reflection emerging as relatively recent features. Given the vast complexity of science and the staggeringly impressive way in which it enables us to both understand and change things in the world, the most simple explanation of why this is the case and how it came to be, is that in its material facet, the world is as science says it is. This however leaves two areas of life and experience unexplained. The first is, what is the status of the apparatus of mathematics which we use to understand the world? Do numbers exist out there in reality? Or are they nothing but constructs that we have invented and which we project onto the world? If this latter is the case then how is possible that we know anything about the world? Also unexplained is the place and status of our conscious thoughts and feelings. Do they really exist and if so how do they fit in with the physical explanation of what a human being is that science delivers? While these are difficult questions, their difficulty does not mean that they are unanswerable or that a pragmatic acceptance of what science says of the material world should immediately be abandoned. Rather the two questions simply imply that there is work to do! Beginning with the nature of mathematics and the status of numbers enables a position to be formulated from which, the beginnings of an answer to the second question may be contemplated and then begun.

During the Middle Ages an abacus was used by merchants to perform the basic operations of addition, subtraction, multiplication and division, with numbers being represented by cumbersome Roman numerals. With the Renaissance this changed and Leonardo of Pisa (around 1170-after 1240), who is more usually known as „Fibonacci“, introduced  to the West,  Arabic numerals along with the handy tricks by which the numerals could be combined and manipulated on paper in a ways that made an abacus look clumsy and obsolete. Nevertheless the abacus had its advocates and an illustration of Arithmetic from the period shows the goddess with two mathematicians in front of her, one doing calculations on an abacus, whilst the other uses hand-written symbols on paper.

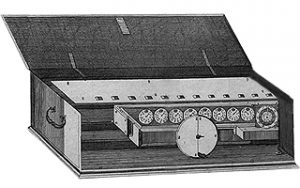
Hidden in this image is the question of whether numbers are nothing but operations of counting, represented, for example, by beads on a wire, or are they invisible entities named by the symbols that represent them? This latter view makes „two“ that which all pairs have in common, „three“ that which all trios have in common, „four“ that which is shared by all quartets and so on. In the „step-reckoner“ or adding machine designed in 1671 by the Baroque philosopher, mathematician and historian, Gottfried Wilhelm  Leibnitz (1646-1716), another question is tacitly raised, for translating the basic operations of arithmetic into systemised, mechanical steps, prompts the question of whether all mathematics can be so represented and defined.

In his Foundations of Arithmetic published in 1884, Gottlob Frege (1848-1925) showed that natural numbers could be defined in terms of classes so that the number two was the class of pairs, the number three the class of trios, et cetera. As classes could be defined in terms of logic, this suggested  that the entire apparatus of mathematics could really be defined in this way. Setting about the monumental task of deducing from a limited number of logical axioms, the truths of mathematics, Frege worked for nine years on the first volume of his The Fundamental Laws of Arithmetic. This was printed in 1893, with the second volume appearing in 1903. Yet just as this second volume was going to press, Frege received a letter from Bertrand Russell (1872-1970), informing him of a paradox. This compelled Frege to add a postscript in which he admitted (1903, p. 253):

For a scientific writer there is nothing worse that can happen than that after finishing a work, its foundations should be shaken. A letter by Mr. Bertrand Russell put me in this position just as this volume was in the last stages of being printed.

Nevertheless after showing how the paradox, now known as „Russell’s Paradox“, could be constructed within his system (1903, p. 253-266), Frege concluded:

The fundamental problem of arithmetic is: how are we to understand logical objects, in particular number? Although this problem has not been solved to the extent that I thought it was whilst compiling the present volume, I am nevertheless in no doubt that the way forward has been identified.

Introducing a rule that outlawed the  paradox,  Bertrand Russell and Alfred North Whitehead (1861-1947) went on to develop an axiomatic system from which it was possible, without paradoxes and contradictions, to deduce the entire apparatus of higher mathematics. Complete with proofs, their three volume Principea Mathematica, was printed between 1910 and 1913.

In terms of logic, statements concerning an object that is present and of which a subject is making some form of claim, take the logical form of „there is an object x such that predicates … hold true of it“. The list of predicates that are true of the object concerned is then listed. In this way we can describe things that do exist such as tables and chairs, friends and relations as well as objects that do not exist, such as unicorns and mermaids.

This method of breaking down and analysing out the import of what we say is the beginning of what is known as „Russell’s Theory of Descriptions“ and was important to Russell as he wanted to show that we can talk about things that may exist without, by the very act of imagining them, implying that they do exist and it was in process of ironing out the anomalies that can come into being when we talk about unicorns and other fictional entities that Russell stumbled on the paradox that bears his name. When we speak of unicorns and mermaids there is a lot more going on than meets the eye. In logic this shows itself via the notion of a class or set of things. Two things belong to the same class when they are in a certain respect similar, in other words two inanimate objects with four legs that support a flat surface, are tables and belong, along with all other tables in the world, to the class of tables, with their „tableness“ being what makes them similar. The notion of classes is of utmost importance for the Theory of Descriptions as in order to say that unicorns do not exist, it is necessary to define the class of unicorns and then to say that the class of unicorns has no members. Thus where subject/predicate logic says „there is a physical object x such that …“, the same thing stated using the logic of class membership says „there is a type of logical object x such that … and the object x is called … and the following objects are members of it“. In discussing things that do not exist reference is made to the similarities in the world that do exist. While unicorns and mermaids do not exist, the animals out of which they are formed as composites do exist.

Thus there are horses and narwhals and goats and also classes of horses and narwhals and goats. Likewise horses and narwhals and goats can be defined in terms of their characteristics, such as having a mane, four legs a beard and a horn et cetera. These components in turn can be defined in terms of other things and properties so that the description of under what circumstances it is that a creature is called a horse, or a narwhal, or a mermaid gets ever more long-winded and unwieldy. The point is that everything we say about the world is done with reference to the similarities that the predicates we use tacitly assume. In terms of class membership, when we say that object „a“ is similar to object „b“, what we are saying is that objects a and b are both members of the same class and partake of the similarity that membership of the class concerned implies. In logic this is expressed by writing:

(E x) (y): y ‚ x

which is shorthand for:

there is an object „x“ and an object „y“ which are such that y is a member of x

This shows that as something that each individual member of a class partakes of, the class itself is in some way independent of its members, which are liable to fluctuate in number. Thus in the above, object „x“ can only be a logical object and the admission of logical objects into the position denoted by the brackets means that they are accorded some kind of existential status. This is to say that, given the existence of horses, goats and narwhals, there is such a thing as the class of unicorns even though the class itself being a so-called „empty set“, is devoid of members. Russell was more than aware of these ontological implications but viewed the inclusion of logical objects into the quantifying position of logical formulas as an „as if“ trick which though it looked as if it made an existential claim, in fact did not. The reason for this was that for a logical system to be able to produce the truths of higher mathematics, it is essential that classes be accorded some kind of existence as the definitions necessary cannot be expressed as the system does not recognise them as valid. Russell’s unwillingness to accord classes any form of existence arose from his stumbling on the at first sight innocuous „class of classes that are not members of themselves“. As a sphere is a physical object, the class of spheres is not a member of itself for it is a logical object and is not something that can occupy space. Yet as Russell realised, the class of classes that are not members of themselves is a member of itself if it is not and is not a member of itself if it is and it was this class that upset Frege’s system. In Principea Mathematica, the class of classes that are not members of themselves is prohibited by Russell’s Theory of Types which with respect to class logic outlaws the formulation of self-referential expressions. For Russell the whole of mathematics was thus an as if trick that only by chance and „somehow or other“ had any connection and relation to reality. Following the publication of Principea Mathematica, the work of Russell and Whitehead was continued by others, including Willard van Orman Quine (1908-2000). Unlike Russell, Quine argued that saying „there is a class of thing x such that …“, is just as much an ontological statement as saying „there is a physical object x such that …“ and that this commits one to accepting that both forms of entity, the physical and the logical, exist. Ultimately this position draws upon and admits that the similarity that we perceive in the world at large does appear to exist and so has no qualms about according classes an existential status. This position is seen as being equivalent to the position adopted by Plato who saw the „tableness“ of a table as an ideal, universal table that existed independently of any particular table and which in Plato’s metaphysics provided a form of template from all the tables that did exist were made. For this reason this view is often described as „Platonic“. Like Quine, the Czech mathematician, Kurt Gödel (1906-1978) also saw mathematical objects in an analogous way to Plato and accorded them an existence that was unrelated to human activity and humans doing mathematics. This means that our mathematical intuition is a response and an adaptation to the world and not an invented construction that has no counterpart in external reality. For Gödel:

But despite their remoteness from sense experience, we do have something like a perception also of the objects of set theory, as is seen from the fact that the axioms force themselves upon us as being true. I don’t see any reason why we should have less confidence in this kind of perception, i.e. in mathematical intuition, than in sense perception.

A little later he says:

Evidently the „given“ underlying mathematics is closely related to the abstract elements contained in our empirical ideas. It by no means follows, however, that the data of this second kind, because it cannot be associated with actions of certain things upon our sense organs, is purely subjective, … Rather they, too, may represent an aspect of objective reality, but, as opposed to the sensations, their presence in us may be due to another kind of relationship between ourselves and reality.

This „other kind of relationship“ is the vast heritage of behavioral responses that we genetically inherit and learn in the womb and during our first years of life and it is this that makes the axioms of geometry and set theory „force“ themselves upon us as being intuitively true. For David Hume however (1711-1776), the similarities in nature that we perceive were inferred by subjects in a manner that was purely inductive, via a step by step process that was based solely on observation. Yet as Russell himself later showed, this is however only half the story for „seeing“ involves assuming that there is and will be similarity in the world. Rather than passively acquiring the habit of perceiving similarities in the world, we actively seek them out and build our conception of the world out of the similarities that we have in part actively projected onto the world. We see similarity in the world because we have to. Nevertheless if there were no similarly in the world we would not be able to make either head nor tail of it. Thus although there must be similarity „out there“ in the world, in any particular instance of seeing and understanding, the similarity that we see is something that we have assumed. This was the conclusion reached by Russell in his book, Human Knowledge (1948). Published after a lifetime of investigating and trying to identify what it is that our experience and knowledge of the world is based upon, Human Knowledge was the closest that Russell got to writing a systematic account of the world. At the end of the work he pragmatically concludes that all human knowledge is based on the assumption of there being some form of similarity that exists „out there“ in the world although there is no logical law that stipulates that this is and must be the case. Nevertheless, though we believe blindly and must believe blindly, there is still justification in assuming that the world at large has organised this state of affairs so that it is to our advantage. In logic this results in a platonic position in which the existence of classes is asserted and the entities of mathematics are seen as objective reflections of an aspect of external reality. It is thus possible to to embrace a platonic position which is ultimately based on a pragmatic belief that similarity exists „out there“ in the world.

Despite the systematic rigor with which the axioms, theorems and proofs of Principea Mathematica were expounded, fundamental questions still remained. Was mathematics an objective facet of reality or was it nothing but a set of rules invented by humans who then devised ways by which their rules could be applied to the physical world in which they found themselves? Were the theorems derived in Principea Mathematica all the truths that mathematics had to offer, or were there other unprovable truths that could be formulated but not proven? Here Gödel found a way of reformulating Russell’s paradox that amounted to the statement:

The class of all statements which are free of contradiction contains a class of statements which are either free of contradiction and are unprovable or are provable but contradictory.

As the contradictions mentioned in the last phrase would render any such system contradictory, “Gödel’s Theorem” is a definite proof that mathematics is incomplete. This means that in any complex system of axiomatic logic such as Principea Mathematica, there will always be mathematical truths which whilst they can be expressed by the symbols of the system and are true, can never be derived or proven using the proofs that follow from the axioms of the system. In addition to establishing that mathematics was incomplete, Gödel also showed that no mathematical system can prove that it itself is free of contradiction. Though there remained the possibility that provable statements might somehow be distinguishable from unprovable ones, this was disproved independently by the American, Alonzo Church and by the British mathematician, Alan Turing. In all of these works, concerning what can be proved and disproved in mathematics, what was assumed and shown, was that mathematics is a symbolic activity. This means that even proofs and theorems that do not consist of numbers, can be redefined in ways which enable them, on a higher level, to be symbolised by numbers. This makes all mathematical truths expressible by such systems, a question of numbers. With mathematical truths and their proofs becoming a question of numbers, the way is opened for the establishing of mathematical truth to become a question of computing and in considering the decide-ability of mathematical truths, Turing postulated „a universal machine“ capable of carrying out mathematical operations. This he envisaged as being able to read and write binary notation, so that equipped with bands of tape, it could be read, write, erase and move over sequences and stop at certain points. Like a typewriter that can shift between upper case and lower case letters, the machine could also shift between different modes so as to perform different operations. With respect to the decide-ability of mathematical statements, which is to say the numbers encoding such statements, in their articles, both Church and Turing used what is known as the „diagonal argument“ developed by Georg Cantor (1845-1918). This Cantor had developed to show that mathematically, there are infinite numbers of infinite collections. The demonstration consists in making a theoretical list of all rational numbers between 0 and 1. Omitting the fractions with cancelling factors this results in a list that begins:

1/2, 1/3, 1/4, 2/3, 1/5, 2/5, 3/4, 1/7, 3/5, 1/8, 2/7, 4/5, 1/9, 3/7, 1/10 …

If these are expressed as infinite decimals the list becomes:

1                                  0.5000000000000000000000000 …

2                                  0.3333333333333333333333333…

3                                  0.2500000000000000000000000 …

4                                  0.6666666666666666666666666 …

5                                  0.2000000000000000000000000 …

6                                  0.1666666666666666666666666 …

7                                  0.4000000000000000000000000 …

8                                  0.7500000000000000000000000 …

9                                  0.1428571428571428571248571 …

10                                0.6000000000000000000000000 …

11                                0.1250000000000000000000000 …

12                                0.2857142857142857142857142 …

13                                0.8000000000000000000000000 …

14                                0.1111111111111111111111111 …

15                                0.4285714285714285714285714 …

16                                0.1000000000000000000000000 ..

17                                …

18                                …

From this list, Cantor then took the diagonal number: 0.5306060020040180 … and systematically changed each digit, by for example, adding one and changing any nines encountered to zeros. In the case of adding one to the above diagonal number, the number that results is: 0.6417171131151291 … This cannot itself be a rational number as at the first decimal place it differs from the first listed rational number by one. At the second decimal place it also differs from the second rational number by one. From any other chosen number, x, it likewise differs, at the x^th decimal place by one. As the list contains all rational numbers, the number thus generated cannot be rational. Moreover any such list can always be used to serve as a means by which another number, not on the list, may generated. Accordingly, there are Cantor argued, more irrational numbers than the already infinite number of rationals (for more on this, see the 2024, Week 4 blog entry, which features an article On Cantor’s Continuity Problem). In his On Computable Numbers of 1938, Turing generated numbers that represented the decide-ability of mathematical propositions including some produced by the diagonal argument. He then showed that the diagonal method could not be mechanised. The numbers generated by the argument were therefore beyond reach of the system. Yet as the machine was a universal machine, this was a general proof of undecide-ablity within all systems. While the incompleteness of mathematics, which is to say of systems of symbolic mathematics, is sometimes presented as if it were some sort of tragic loss there is no reason to see it this way. Rather it simply shows that whilst one can divide the world into parts and extract a system of mathematics that deals with the relationships pertaining between such parts, there will always be something indeterminate that, lying out-with the confines of whatever system one has chosen to set up, will accordingly be unpredictable. Philosophically this implies that whilst it is not inappropriate to divide the world up, what is learned as a result, will not and never can be, the whole truth. Not dividing the world up into parts that can be investigated means that we have nothing to say about the world as, as seen, language works by seeing the world as being composed of parts about which things are said due to some parts being similar in certain respects to other parts. For Gödel:

The set-theoretical paradoxes are hardly any more troublesome than for mathematics than deceptions of the senses are for physics.

By this, Gödel means that paradoxes such as Russell’s paradox are similar to phenomena in the physical world such as a stick that inserted into water appears as if bent at the water’s surface or in a desert, the phenomenon of a mirage. Published in 1931, only eight years later, Gödel’s „Incompleteness Theorem“ and the ardently held debates on the nature of mathematics, suddenly acquired a practical relevance that soon proved to play a not insignificant part in altering the course of the Second World War. As radio contact was quickly found to be essential for modern warfare, the perennial problem was of how to make messages incomprehendable to all but the intended recipients. In 1918, the electro-technician, Arthur Scheribus had devised an encryption procedure that in 1926 had been used for military purposes and which, following Hilter’s rise to power was successively improved so that by the outbreak of the war, its use was an integral to military and diplomatic communication. The revised device was the celebrated „Enigma“, whose encrypted messages were believed by the Germans to be uncrackable.

Although „Turing’s „Universal Machine“ was hypothetical, its principles formed the basis for his crypto-analytic work at Bletchley Park where in 1939, he completed the design for a computing device that systematically searched through the encryption possibilities of Enigma encoded messages. Known as a „Bombe“, when in operation the machine made a ticking noise reminiscent of the time fuse on an explosive bomb.

Although Turing’s Bombes could work much faster than any human operator, speed was nevertheless only half the secret for had the Bombes worked systematically through every available possibility, the intercepted messages would still have taken so long to be deciphered that the advantage gained by knowing the message’s content would be lost or significantly offset by its being out of date. Yet from the investigations into the foundations of mathematics carried out before the war, Turing knew that in formal systems, truth could be acquired at the expense of certainty and completeness. Proceeding step-by-step in search of a set of encryption settings that was certain, was similar to wanting a step-by-step proof in mathematics. If the demand for perfectly justified methodical steps was relaxed or abandoned, large numbers of unlikely alternatives could be eliminated leaving a much reduced number of more likely combinations which the Bombes could work through in a time that did not overtly comprise the actuality of the information. In this way, through the prompt decipherment of information at Bletchley Park, Turing and his colleagues are reckoned to have knocked two years off the length of the war.

In Russell’s Theory of Types and the other rules according to which classes may be defined and postulated are such that while things exist and may be said to exist, existence itself is not a predicate but rather is an operative symbol and states of affairs in the world either exist or do not exist and so either are or are not. This means that at the level of classes, existence is not something that can be talked about. This is because it cannot be inserted into the quantifier position of a logical statement, as it is not an object nor can it be put into the predicate position, as it is not an adjective. At first sight this makes it look as if the existence of God is not something that can be proved as traditional proofs of God involve saying that God is good and God is great and that in order to be perfectly good and perfectly great, he must also exist as otherwise he would be imperfect. God’s existence is thus something that is necessary with „existence“ being something of which it is maintained that the predicate „is necessary“ holds true of, with it being however precisely this that class logic outlaws. Yet just as Gödel’s Incompleteness Theorem re-introduces Russell’s Paradox by, instead of talking about classes, talking about classes of statements that are true, provable or un-provable, so too Gödel saw that the old arguments concerning the necessary existence of God could be re-introduced with renewed validity. Like Russell, Gödel saw the similarity and continuity that we encounter in the world and as essential for our survival. Moreover, as observed by the Austro-British philosopher Sir Karl Popper (1902-1994), for any problem there are always good and bad solutions and as all life-forms, as long as they live, are engaged in the solving of problems, this gives an objective basis by which the concepts of good and bad can be defined. This in turn makes the preserving of the similarity and continuity that characterises the world, integral to any conception of the Good. Reflecting this, with reference to his proof, in his philosophical notes Gödel wrote:

The fundamental philosophical term is cause. This involves: will, power, pleasure, God, time, space. The affirmation of existence is the cause of the world. The first being: to existence, there comes an affirmation of existence. From this it further follows that as much existence as possible should evolve – and this is the ultimate reason for diversity (variato delectat).

Harmony entails more existence than disharmony, for the opposites negate their existence. Regularity consists in agreement, for example the same angle, the same colour.

Variato delectate means “variety delights”. Today, life on Earth features an unparalleled range of diversity that consists of some 5 to 9 million different species. The ways in which animals, birds, fishes and insects interact both with each other and with the rest of the environment are highly complex and only partially understood. Although we are often unaware of it, many of these interactions are such as to provide invaluable services to mankind and the stability of the natural world thus constitutes an immense package of similarity and continuity on which human civilisation is completely and utterly dependent. Never publishing his proof, Gödel shared it in 1970 during a period of illness from which he thought that he might not recover. His reluctance to publish was due to his not wanting it to be seen in the same light as traditional proofs. Rather he wanted it to be seen as part of an apparatus of speculation that arises whenever we begin to think seriously about the world. In view of the fundamentally essential role of similarity and continuity in human life and thought, considering what is likely to happen when the eco-systems of the world get out of hand is not only topical but gives Gödel’s proof a new measure of relevance when we consider that without the Good as conceived of by Gödel, life as we know it would collapse. An honest and informed striving for a good that is beneficial for all people both now and in the future is essential for our survival it and moreover automatically prescribes an environmentally responsible mode of conduct as everything we do has implications for the environment and for those who come after us. This however raises questions about how are we as individuals to know what is good and what is not good. In striving for the Good what is permissible and what is not and who i) decides and who ii) is responsible when well-meant plans go wrong?

For any deed undertaken or any mode of conduct lived out there is always the danger of it being overtaken by events and contexts whose complexity and knock-on effects we fail to apprehend. The immense and profound gravity of this problem was considered by Dietrich Bonhöffer (1906-1945), who was a priest and objector to the Nazi regime. Banned first from speaking and then from writing, Bonhöffer saw that in a regime such as Hilter’s, pacifism, by virtue of not rocking the boat and not presenting the system with any significant problems, over time, was indistinguishable from someone who, by merely „doing their duty“, simply wanted to get by without killing anyone and without being killed and apart from not out-rightly embracing Nazi ideology did not otherwise undertake take any form of moral stand. Bonhöffer therefore came to the conclusion that in such a case focused violence was the only option available. Yet here a host of difficult questions arise. In making an effective act of resistance is it justifiable to kill and if so, is the unintended killing of innocent people also justifiable? In cases where action taken resulted in the unintentional killing of innocent people, Bonhöffer saw the crippling guilt that could be engendered, as something that could be overcome through trust in the all-forgiving Grace of God. This and the reconciliation that God initiates between man and himself, he saw as being rooted, not in Christ’s death upon the cross but rather in God’s decision to become human and descend to Earth in the first place. Taking this thinking further, one can observe that, as only a human being who has been wronged can forgive a human being who has done wrong, it is for this reason that God must be thought of as sending himself, in the form of his only Son, down into the world to take upon himself the sins of the world so that those who have sinned can be forgiven by him on a human, one to one, personal basis. Repeating the pattern set up by Gödel in his Ontological Proof, this observation can be rendered in terms that make it necessary, so that if the Good is sought, there is of necessity a God and of necessity there is forgiveness and accordingly, in some sense, a human manifestation of the Divine that enables forgiveness to be granted, as this is the only way that human beings who have done wrong can receive forgiveness and experience the absolution of their guilt. Here the pivotal realisation is that in spite of all the science and mathematics we have at our disposal, we are emotional beings and if we are to have a future, we must re-define ourselves both emotionally and intellectually and on both levels re-establish a relationship and rapport with that which lies over and above us. Despite his being forbidden to write, from 1940-1943 Bonhöffer worked on a book of ethics and theology in which he intended to provide a new theology for the Germany that would come into being once the war had ended and the dictator had been removed. Becoming involved in a resistance group and knowing that he might at any time be arrested, Bonhöffer hid the various parts of his writings in his garden, whilst other parts, although confiscated by the Gestapo nevertheless survived. Early on in the work, Bonhöffer diagnosed the nothingness into which he saw the western world as falling:

This nothingness into which the West is sliding is not the natural end, the dying, the sinking of a flourishing community of peoples. Instead it is again a specifically western nothingness: a nothingness that is rebellious, violent, anti-God, and anti-human. Breaking away from all that is established, it is the utmost manifestation of the forces opposed to God. It is nothingness as God; no one knows its goal or its measure. Its rule is absolute. It is a creative nothingness that blows its anti-God breath into all that exists, creates the illusion of waking it to new life, and at the same time sucks out its true essence until it soon disintegrates into an empty husk and is discarded. Life, history, family, people, language, faith – the list could go on forever because nothingness spares nothing – all fall victim to nothingness.

Gödel’s Ontological Proof puts this insidious all-consuming nothingness into context when one  realises that modernism must embrace a doctrine of nothingness as otherwise it would be forced to acknowledge God and this goes against the modernist mantra of „… but we know better“. The nothingness identified by Bonhöffer is driven by the knowledge that anything we make will soon enough be produced more cheaply by someone working with an improved machine or by machines working 24 hours a day by themselves. This has lead to a culture of cynicism and an attitude in which anything that strives for value is dismissed. By not rocking the boat and not posing any fundamental challenges, all this supports the modern world’s, at times tacit and at times blatently declared credo that the point of life is to make money, this in turn fueling the gnawing doubt identified above, that lies at the heart of capitalism. The relevance of Bonhöffer’s message is that the credo that the point of life is to make money, is a godless derivative of the protestant low church attitude to life. In protestant low churches, the tacit implication is that the point of life is to live in a manner that will ensure that one is admitted to heaven. This makes life a test, with the joys of this life being but a passing irrelevance. In like manner, according to Neo-Liberalism and the Homo oeconomicus view of what a human being is, life is about earning and spending money and nothing else. In this view, which in its simplification can be seen as being descended from the low church view, God is rejected and heaven is replaced with the idea of money. In this context, Bonhöffer’s theology is important as it explicitly denies that life and the human body are merely the means by which the test of a person’s worthyness for admittance into the Kingdom of Heaven is conducted. Rather life and the human body are ends in themselves and this means the joys of life are the joys of God and as such are to be cherished and enjoyed both in the present and as memories. A Lutheran, Bonhoffer’s Ethics provides a fully worked out means by which the low-church attitude to life can be called into question and by implication, the absurdity and utterly unfounded nature of the Homo oeconomicus myth further exposed. For Bonhöffer, the omnipresence of Christ in the world of today was a central truth which he saw as being the departure point for all else. Accordingly the word of God was not „forever“ but rather was ever omnipresent, in the here and now of today. For this reason Bonhöffer saw theology as being perpetually rewritten in order that it speak the language of the present. This however did not mean that compromises had to be made with other forces at large in the world, such as National Socialism. Of particular relevance here, Bonhöffer advocated a „natural life“ in which:

Reason perceives the universal in what is given, as reason perceives it; is also something universal. It embraces the whole of human nature. Reason recognises the natural as the given, as what is universally established, independently of being able to prove it empirically.

A decisive consequence follows from this. The natural can never be a construct of some part or some authority in the fallen world. Neither the individual nor any community or institution in the preserved world can set and decide what is natural.

Inherent in the Natural is an acceptance that things can be ends in themselves and as shall be shown, this is inherent in chaos theory, in which from out of chaos, order emerges and enables the coming into being of further forms of order of ever growing complexity and intricacy. Inherent in this is the fact that all things are interrelated, for each layer of order perceived rests upon an underlying layer.

In seeking a way of thinking about the Divine that is scientifically grounded, explains phenomena and yet is also humble and does not dogmatically deny the existence of that which it cannot explain, it is instructive to return to a metaphor for God developed by Nicholas of Cues (1401-1464), otherwise known as Cusanus. This provides a means by which the coming into being of a new world can be followed, so that from out of the disorientation, doubt and chaos that the nothingness identified by Bonhöffer leaves behind it, a new view of life and the world can be brought into being. With a single sweep, a circle can embrace all that lies within its radius, whereas straight lines can never do this but rather can only enclose an area when instead of there being but one straight line there are three. Traditionally therefore the circle and sphere are associated with the divine, whilst straight lines, along with the figures and solids that can be made from them, are seen as earthly. Where straight lines are easily measured, all that is circular is elusive and somehow otherworldly and in order to quantify something that is circular or curved, reference must be made to π. Given a right-angled triangle, the length of whose lesser sides are given by whole numbers, the length of the hypotenuse is given, either by a whole number (as in a 3,4,5 triangle) or by an irrational root (as in a 1, 2, √5 triangle or a 1, 1, √2 triangle). Although irrational and with a process of enumeration that goes on forever, these irrational’s and a host of associated irrational numbers, such as phi, which characterises the Golden Section and towards which the Fibonacci sequence tends, are nevertheless not irrational in the way that π is. This was first realised by Cusanus who saw that π is what mathematicians now call a „transcendental number“. This means that the irrationality of π and other transcendental numbers is different from and transcends the irrationality associated with the diagonals of geometric figures. In what mathematicians call a polynomial equation, rational and irrational numbers can be characterised by means of whole numbers that reduce the number concerned to zero. Thus where √2 is defined as x² – 2 = 0, transcendental numbers cannot be characterised in this way and are seen as belonging to a number class of their own. For Cusanus, no earthly square could ever be equated with the insensible perfection of a circle. The wish Cusanus said, for there to be such an equality was a result of our seeing things with mental eyes so that, apprehending the divine, we were inclined to forget the limitations of the corporal world. Cusanus saw the differences between rational and irrational numbers as indicating something of the differences between our human powers of rationality and the all-encompassing, ineffable nature of God. For Cusanus, God could not be known or apprehended through rational means but only once rationality had been dissolved and it had been accepted that when it comes to God and the nature of God, we known nothing. This recognition however enabled God to be approached by other means. The purpose of philosophy was therefore to show that when it comes to God, we know nothing. Likewise, in mathematics, where rational numbers reflected the reality of the material world, irrational numbers, by showing the shortcomings of our day to day rationalism, pointed to the divine. In this way Cusanus saw Christ’s earthly nature as melting into the eternal circle of his divine nature, in exactly the same way that an infinite polygon fades into a circle. This may be shown when the lengths of the sides of the regular plane figures contained within a single circle are divided by the diameter of the circle and plotted on a graph. As the number of sides increases and the lengths of the regular figures get shorter, the points plotted out approximately follow a curve known as a tractrix.

In order to escape the modernist void of nothingness described by Bonhöffer and see how things can be ends in themselves, it is instructive to imagine a rotating disc. This has a smaller disc is mounted at a point one radius length in from its circumference and when as the larger disc moves, the smaller disc rotates in the same direction. With this simple apparatus a series of thought experiments can then be conducted.

Experiment 1

If the two discs both rotate at the same speed and so execute the same number of rotations in a given period of time, they will be synchronised and a particular point on the smaller disc will, after one revolution, meet up again with a particular point on the larger disc. If the point on the smaller disc lights up whenever it brushes with the circumference of the larger disc then the impulse of light will always be at the same place. Taking this point as defining 0°, if the speed of rotation of the smaller disc is increased then the light will no longer light up at 0°, for with each revolution of the larger disc, the smaller disc will complete more than one revolution so that the flashing light will move around the circumference of the larger disc. As the speed of rotation is increased, this movement around the disc will get faster and faster. At certain point the light will return to the 0° point and if acceleration is stopped, will remain there emitting a rhythmic pulse. 180° away from zero it will also be flashing rhythmically and from this it can be deduced that the speed of rotation of the smaller disc is twice that  of the speed of the larger disc. Progressively increasing the speed of the smaller disc will result in the same phenomena occurring again, only when the light returns to 0°, instead of there being one other stable point at which the light flashes, there will be two, each 120° away from 0°. This can be carried on until the number of stable points is infinite and instead of there being flashing lights, the arc of a circle moves around the circumference, the length of the arc being related to the glow time of the light. This thought experiment shows how the whole numbers of the ratios 1:1, 1:2, 1:3, 1:4, et cetera that characterise the rotations of the smaller disc relative to the larger disc, act as what mathematicians call „strange attractors“ – points of stability that arise in what is otherwise a sea of perpetual change.

Experiment 2

While Experiment 1 is so straight-forward as to be almost banal, it nevertheless introduces the concept of a strange attractor and sets the stage for a scenario in which the unexpected comes to the fore and the predictably straightforward world of conventional mechanics is by-passed. If the larger disc is said to react to the increasing speed of the smaller disc and following a certain delay, to likewise increase in speed, then the situation becomes complex with the two discs behaving as if in a race with each other with victory frustratingly slipping out of sight once the lead has been gained. For although the smaller disc can always go faster than the lager disc, the larger disc can always catch up with it. This makes the increased speed of the smaller disc relative to the larger, something that continually grows and then diminishes. On a graph known as a logistic parabola, this can be shown by plotting the increase in speed of the smaller disc is plotted against the period of time over which it is accelerated and made to rotate faster and faster. Although the acceleration of the larger disc is not shown, its effects on the speed of the smaller disc are. On the logistic parabola, the parameters are rigged so that the graph is a square in which the curve begins and ends at zero and on the y-axis the maximum attains a value less than 1. As the smaller disc is accelerated, compared to the larger disc it completes ever more rotations, so the curve rises steeply upwards. Then, as the larger disc begins to accelerate and likewise completes ever more revolutions, so this steeply rising curve flattens out and the maximum is reached. With this, the smaller disc is no longer rotating faster than the larger disc and the difference in speed begins to diminish. Although the speed of the smaller disc is still being progressively increased, that of the larger disc is also increasing and as the cycle of the larger disc is behind that of the smaller, the larger disc will, from this point on, always be going faster than the smaller disc and so the difference in speeds between the two will always be getting smaller until the two are both going at the same speed (this being of course a much increased speed compared to the speed than when the was experiment started). As in Experiment 1, there are stable points, the first of which is to be found shortly after the curve reaches its maximum. Here, at x = x*, if acceleration is stopped, a stable situation will result with the light on the smaller disc always flashing at the same point.

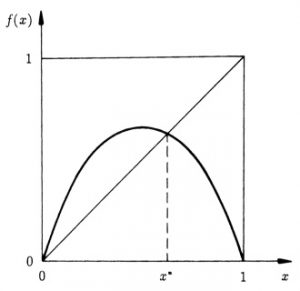
This situation is usually introduced with reference to a population of rabbits that is preyed upon by a population of foxes. As the rabbits increase, so too does the number of foxes that prey on them until there are so many foxes that the number of rabbits begins to fall. There then comes a point when there are more foxes than can be sustained by the rabbit population. The number of foxes therefore declines, which with a delay factor, enables the rabbit population to grow again. The next year there are more rabbits and the number of foxes again begins to increase until the population is once again decimated where upon the foxes again find themselves without food. If these ups and downs are plotted on a logistic parabola, the curve that represents the rabbit population rises upwards until it reaches a maximum. Then at x*, a stable population results, preyed upon by a likewise stable population of foxes. If however, the rate at which the rabbits reproduce continues to increase, or, returning to the case of the two discs, the smaller disc is accelerated further, then f(x*), the stable point that is associated with x*, will become unstable and dissolve. In the case of the rabbits, the population decreases, in the case of the two discs, the difference in rotational speed lessens until at x = 1, there is no difference and despite the increased rate of reproduction, in the case of the rabbits, there are no more rabbits.

Experiment 3

In order to see what happens when the situation outlined in Experiment 2 is continued and the system is subjected to further acceleration or an increased rate of growth, the f(x) values from Experiment 2 are taken and are fed back into the function to get f²(x) values. On the lower of the two graphs shown below it can be seen that instead of one maximum there will two, separated from each other by a trough. After the trough and before the second maximum, the increasing rate of growth leads to the curve rising upwards in a way that is formed from two scaled rotations of the curve on the first graph, at the point where, after the maximum was reached, the curve began to dip down towards f(x*). Here the scaling factor is 2.618… or phi + 1, where phi is the number associated with the Golden Mean.

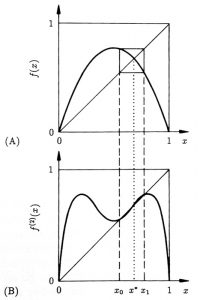
As each of the two segments of the new curve is similar to the original segment, the same dynamics apply but at at two points on the curve instead of one. Thus f(x*), which was once an attractor, becomes instead a repellor and pushes the values of f(x) either down to f(x₀) or up towards f(x₁), so that f(x₀) and f(x₁) become the new attractors and the new stable or fixed points of the system. In the case of foxes and rabbits, this corresponds to a case where, due to an increase in the breeding rate of the rabbits, the once stable population at f(x*) has dissolved and instead there is a maximum at f(x₁) and minimum at f(x₀) with the population oscillating dynamically between the two extremes.

Experiments 4, 5, 6, …

To see what happens when acceleration is continued, the results of Experiment 3 are fed into the f(x) function to result in f³(x) and the same can be done repeatedly to result in f⁴(x), f⁵(x), et cetera. In each case, the number of stable points in the system, which in chaos theory are known as „orbits“, doubles, so that the number of orbits generated by f(x), f²(x), f³(x), f⁴(x), f⁵(x) is 1, 2, 4, 8, 16, et cetera. This form of what mathematicians call „bifurcation“ is known as „period doubling“. On the logistic parabola, another form of what mathematicians call „bifurcation“, is „period-trebling“ in which stable orbits of 3, 9, 27, et cetera are generated. The phenomena can however also produce five-fold, seven and nine-fold bifurcation’s. Returning to the rotating disc, this means that as the two discs are progressively accelerated the stable orbits that arise are those of the number series 1, 2, 3, 4, 5, 6, et cetera and from the points where the light repeatedly shines at the same place, triangles, squares, pentagons, hexagons et cetera can be constructed. Continued ad infinitum the process of drawing plane figures with ever more sides results in a circle. In this way, Cusanus‘ metaphors can be resurrected, with the tractrix that lies behind them being integral to the process.

A work by Alexander Curtis in which life, death, ignorance, knowledge and the beyond are thematisied in a manner that is tune with Cusanus‘ thinking. Formally the departure point of the work is a blackboard

If on the logistic parabola, the scaling factors are compared from one graph to the next it will be seen that they rapidly tend  towards a constant of 2.5029…, which known as alpha,  is integral to chaos theory. The point at which bifurcation results in an infinite number of stable orbits, is chaos as instead of having a finite number of stable orbits, or fixed points between which the system oscillates, there are an infinite number. In a chaotic state, while a system is recognisable and has its usual form, aspects of its behavior are unpredictable. Once a chaotic state has been reached, if the rate of growth or acceleration is increased still further, then it will be found that from out of the chaos, a new series of stable orbits will arise, exactly as before and these again can be increased and likewise, from them, a new state of chaos brought forth out of which further stable orbits can be made to arise. In this way, from out of chaos, order can arise and equally, chaos can be made to reappear and this branch of mathematics is known as chaos theory. This shows how behind the supposedly staid and solid strange attractors of Experiment 1, there is whole world of dynamic interactions in which systems no longer behave in a linear fashion. As stable states of affairs in the world are equivalent to stable orbits, this moreover provides a theoretical demonstration that things can be ends in themselves.

To relate these experiments to the world of space and time in a literal way, the experiments above are repeated with the revolutions of the points around the circumferences of the two discs being shown as sine curves. Where a circle shows the route taken by a point as it moves around the circumference, a sine curve shows how the point oscillates away from and then towards a straight running through the centre of a circle. By virtue of this repetitive movement the points on the line can be seen as representing successive moments in time that visually the sine curve represents the rotational movement of a point along a time axis, t.

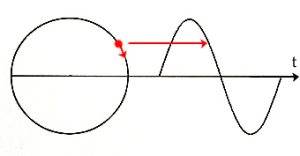
If as in the above experiments, the  point rotates continually around the circumference, the result is an endlessly undulating wave. Depending on the size of the circle and its speed of rotation, sine curves can be of different amplitude and can have different frequencies. The different curves can however be added together to create a complex curve, which can at any time be broken back down into its component curves. In the graph below the three monotone curves of different amplitude and frequency have been added together to result in the red curve.

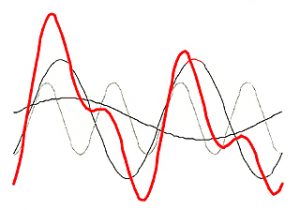
Although the sine functions that characterise sine waves are what mathematicians call „continuous“ and generate curves that extend out to infinity, by a technique which involves considering all possible sine functions, particular functions can be introduced and terminated as required. This means that anything drawn graphically on a graph, regardless of whether it consists of curves, straight lines or combinations of the two, can be defined in terms of sine waves. This is shown in music where any sound imaginable can be synthesised in terms of a varying one-dimensional value and in DVD technology where visual images are reduced to  frequencies that occupy two dimensions. Taken to its most minimal extreme this results in Dirac’s function which at zero is infinite, whilst elsewhere it is zero. In mathematics this way of modelling things by means of sine waves and their functions is called Fourier Analysis and is just as true in three dimensions as it is in one and two. Accordingly, if the above experiments are repeated and from the rotating circles, sine waves are extracted, from the rates of growth that resulted in the formation of stable points, stable sine waves will result with frequencies that are expressible in terms of whole numbers. Using these stable building blocks, anything desired can be constructed so that through the combination of chaos theory and Fourier analysis, a convincing two and three-dimensional account of the universe can be built up with the stable points of chaos theory being that which underwrites the similarity that we encounter in the world.

Going beyond what we see, what is built up can be abstract modelling’s of relationships that pertain between things in the world and which, whilst abstract and unseen, are nevertheless of relevance to life as we know it, with modern science now seeing non-linear dynamics as being everywhere. It is at work at various levels in our bodies and those of all other living things, it is at work in to the dynamic interactions of animal populations with each other and is what lies behind populations of viruses, bacteria and other microbes. Chaos theory governs fluid mechanics and underwrites the stability of our Solar System. Even though it involves only numbers, its applications are so numerous that the constant associated with it, delta, is known as the „universal constant“ with delta being related to the scaling-factor, alpha already encountered. The problem is that when one tries to consider all the components of an eco-system taken as a whole and all interacting at once with each other, the task becomes impossible, as due to the mutual interactions in “fundamentally complex systems”, unpredictability and a degree of indeterminateness are inherent. Fundamentally complex systems can be surprisingly simple and begin with the gravitational interactions of three balls, or as shown below, two weights on a jointed pendulum.

Given a gentle push, the pendulum moves back and forth like a single joined pendulum, yet when a powerful flick is imparted into the system, the motion that results becomes chaotic and unpredictable. The movements of the planets around the sun is similar and whilst not chaotic, is not stable but rather is semi-stable. Once matter has come into being, chaos theory can be rightly said to be that which underlies the development of all that the universe contains. Chaos theory thus enriches and gives new meaning to Cusanus‘ metaphor of God. It also explains and puts into context Plato’s conception of the Same and the Different with which in the Timaeus, the Demiurge begins to fabricate the universe. This is close to the conception of the universe arrived at by modern physics in which, before the big bang, the universe is seen as having been a supra-compressed, supra-hot and supra-ordered mass of unimaginable density whose perfect order was suddenly shattered. Resulting in an exploding universe, the shattering’s ultimate goal is to achieve a state of maximum entropy. Not chaos, entropy is a state in which nothing can happen because everything that could happen, has happened. Although everything in the universe will one day be reduced to a small number of basic particles whose spatial positions and configurations are perpetually changing so that life and continuity will be impossible, as Plato perceived, such a form of perpetual change is also a form of continuity, so that within the Different there is a hidden element of continuity. Equally in the Same there is also an element of difference as in order to manifest itself, Sameness must in some way have different facets which are nevertheless part of a whole. Although it is a fact that one day our universe will become nothing but an evenly mixed collection of basic particles, from a point of view within the universe, this this is not its point. Rather, the point is the journey from a supra-hot and supra-condensed mass towards a state of extruded maximum entropy. Along the way, order and chaos dance around each other, bringing patterns into being that are at times beguilingly simple, at times breathtakingly complex. Like a firework, it is not important where the burnt-out shell of the rocket lands but on how it looks as, once it have been shot up into the air and exploded, it emits a radially expanding pattern of light that glows briefly in the dark. It is this brief glow that is the point of the universe and the point of life. Chaos theory describes the structures of the paths taken by processes in the universe as they develop from a state of maximum order towards a state of maximum entropy. In Plato, the equivalent to chaos theory is the harmonic theory that the Demiurge draws upon when, having made a mixture of the Same and the Different that includes a notion of space,  he divides the mixture up into lengths which are proportionally arranged so that a musically ordered, living universe results. Thus in Plato and in the pragmatically grounded form of Platonism here expounded, from out of life there arises the Good and from out of the Good, there arises a notion of God and the ethic that all life is to be cherished and rejoiced in. Where living in tune with these things is good:

ignoring them and treat them with contempt is bad:

Where a sphere is characterised by a single positive curve of +1, its converse is characterised by a single negative curve. This results in something that, were it to be conceived as a solid, would resemble the spinning top were it not for the fact that only not only are the poles and equator are flung out to infinity.

This means that the „solid“ can never be apprehended as such and indeed it is not a solid in any conventional sense and cannot be accurately represented in three-dimensional space. Although counter-intuitive, the notion of such a surface is not illogical or contradictory and within mathematics has even been proven to exist. Moreover it has the capacity to be all-encompassing in ways that spheres and circles never can be. As the poles and equator are at infinite remove, there is and can be nothing that lies beyond the surface and so there is no „outside“ and the surface is all-containing. From this, it follows that there can be only one surface of constant negative curvature. For a second surface, like the first, being all-containing, would have to differ from the first in a way that lay out-with both surfaces, which, as there is no out-with, is impossible. Self-contained, a sphere is defined in a finite and limited fashion so that when fashioned by hand, the making process can interrupted at any stage and resumed later. Equally, neither a half-made sphere, nor a half-drawn circle will ever finish the making or drawing process themselves. In the case of a surface of constant negative curvature, the curve is self-contained in a way that is unlimited and infinite. This means that instead of being contained by the planes of the space with which it is associated, like spheres and circles are, the curve contains the planes with which it is associated. This in turn means that, as soon as a surface of constant negative curvature has been begun, it comes into being all at once. Thus regardless of on which side of the equator such a projection is begun, the surface is engendered on both sides of the sphere’s equator and reaches both up as well as down and round about. This means that it cannot be correctly imported into three-dimensional space or accurately depicted in two dimensions. In this respect the surface can be seen as sharing a number of aspects in common with that which is generally called „God“. Using a system of projection that relates the circle and the sphere to a surface of constant negative curvature, Alexander Curtis has developed a model of the self in which the gradual development of our sense of self from infancy through childhood, adolescence and into adulthood is explained and put into context. Although explaining the theory goes beyond the bounds of the current essay, this is undertaken in A Theory of Self and Conscious, which is to be made available as a print-on-demand booklet.

In the model, a geometrically derived axis of good and evil is seen as coming into being as we progress from childhood to adolescence. This results in a conception of good, bad and the Divine with this geometrical conception of the Divine being analogous to the logic of Gödel’s Ontological Proof. Moreover the chaos theory that is a characteristic to so much of what takes place within the world, is written into the fabric of the all-encompassing surface of constant negative curvature, suggesting that this is a genuine and natural instance of reason identifying something that is universal and genuinely of the world as opposed to be being something that is artificially projected onto it. Where π is associated with the circle and sphere and thus with the infinitely far removed equator of a surface of constant negative curvature, the number associated with the actual surface of a surface of constant negative curvature derives from a sequence that reveals something of the nature of the curve. The sequence is a binary number and is generated by taking 0 and 1 and inverting them to make 1 and 0. Tagged on to 01, this makes 0110. This is then inverted and tagged on to 0110, to result in 01101001, which in turn is inverted and tagged on to what has already been arrived at. In this way, an infinite sequence is generated, known as the Morse-Thule sequence and which, converted into decimals results in the Morse-Thule constant 0.412…, which is also a transcendental number. The Morse-Thule sequence is related to a surface of constant negative curvature through the fact that although it is generated through a process of systematic repetition that results in units that can be reconstructed, the way that the units reach out to infinity is not periodic and yet, unlike the sequences that make up π, they are nevertheless in their own and possibly unique way, systematic. This means that by systematically using the units of the sequence, a curve of constant negative curvature can be generated. As found by the mathematician Manfred Schroeder, when period doubling is analysed using a technique of symbolic dynamics, the Morse-Thule sequence emerges. Where the circle can be seen as a metaphor for that which is divine and which lies over and above us, chaos theory is the rationale of that which lies enclosed within the circle. Lying all around us and percolating through us, it forms and molds, not only us but the substance of the world in which we live.

It being established that similarity and classes of things can be seen as being existent, it follows that the self as defined according to the model worked out by Alexander Curtis, also exists as long as the biological body associated with it is alive and awake. Nevertheless the stuff out of which consciousness is actually „made of“ is still in need of some form of explanation. When we experience something, whatever we experience is experienced as an experience that is unique to us alone and cannot be directly accessed by anyone else. Although we can share our experiences,  this takes place through our using words to indicate what we have experienced. The words we use name things that, we assume, are the same for others as for ourselves. Thus we assume that what someone else experiences as „hot“, is hot and what others see as being red, is the same as what we call „red“. Here again, there is an assumed similarity that is implicit and it is this assumption and the fact that it is only very rarely contradicted that underwrites language and enables us to share our experiences and the nature of our feelings with other people. In this way, we gloss over the fact that for all a physicist may say about the wavelengths of the light that result in „red“ and all a neurologist may say of neurones and firing synapsess, the experience of seeing red cannot be described by science and can only be named by words. With respect to our feelings, the same is also true only the gulf between what is actually felt and the hormones with which science explains our feelings, is more obvious. In both cases, the glossing over takes place as a result of talking about our inner states in exactly the same way as we talk about states of affairs in the outside world. Using the subject/predicate logic already encountered, we assign predicates to objects and subjects indiscriminately. Thus when talking about our inner states, instead of referring to „object x“ we use the word „I“ and then assign a predicate that names what we have experienced or are experiencing. We thus forget that whilst we can talk about our inner feelings and experiences, we can never take them apart and analyse them like a mechanic when confronted with a device whose purpose is unknown but which can be identified through taking apart. In all that is subjective, there is no potential for things to be taken apart, for taking apart destroys that which would be investigated. This being said, the question, „Out of what sort of stuff might our inner experiences be composed of?“ can still be asked and we are allowed to expect an answer. As the logical objects that reflect the similarity of the world have already been postulated and accepted as existing along with the physical entities that lie behind them, the simplest answer to the question is to say that our conscious experiences are made out of the logical entities that are associated with the physical entities with which consciousness is also associated with, viz. the brain, a body, neurones, et cetera. That thereafter, nothing more can be said is due to the divide that exists between that which is objective and that which is subjective. The explanation is thus restricted to deciding into which ontological catergory of thing the stuff of consciousness is to be thought of as belonging. In this way, whilst associated with states in the brain such as networks of firing synapses, consciousness itself is immaterial and constituted out of the similarities and differences that pertain between the brain and its parts and the brain and the various parts of the body to which it is connected. Whilst this is abstract, it is so by definition and moreover does not involve dogmatically reducing the nature of our inner experiences to purely physical states, as was done for so much of the twentieth century, nor does it involve invoking an otherworldly soul that in an utterly inexplicable manner somehow interacts with the physical body with which it is associated.

Having explained the self and clarified as far as possible out of what it is that the experiences of the self are made, the question of whether the self can in some way survive death remains. While from an atheist point of view, the positions argued for above entail a clear „no“, for the believer and the agnostic, the matter is more complex with factors  in a number of disciplines allowing scope for more subtle interpretations. To this effect, one of a number of projects that Alexander Curtis is saving for a rainy day is an examination of the “theological logic” hidden in the Plato’s notoriously difficult Parmenides and in Ficino’s concept of “Angelic Mind”. Meanwhile in physics, our universe is now seen as being part of a multiverse, in which universes come and go like bubbles of steam in a pot of boiling water. Usually each universe is seen as being separate, self-contained and alienated from any other. Thus in another universe, given that there is such a thing as light, the speed at which it travels will not necessarily the same as in our universe. Yet for the theoretical physicist, Michio Kaku, string theory exists in the multiverse, making it is a possibility that we exist in parallel states (see his books, Parallel Worlds and The God Equation). In tune with a view of the world that ultimately derives from Plato, Kaku sees the final theory of string theory as being „based on music. Music is based on vibrating strings. Each vibration corresponds to a particle, so the electron vibrates this way, a proton vibrates this way and a neutron vibrates this way. There’re nothing but musical notes. So physics is the harmonics that you can create on musical forms. Chemistry is the melodies that you can create out of these musical notes. The universe is a symphony of strings, and then the mind of God, the mind of god that Einstein wrote about for the last thirty years of his life, would be cosmic music resonating through hyperspace.“ In addition, one must accept the latest recognition’s of physics according to which 75% of the „stuff“ of which the universe is made of, is unaccounted for. Of the remaining 25%, 10% is what is already accounted for, whilst 15% is assumed to be such that it will one day be describable by physics if current thinking and investigations are continued. Importantly, this 15%  includes „dark matter“ and „dark energy“. The 75% that is unknown, is thus unknown in a truly humbling, baffling and awe-inspiring way. It is there and yet we haven’t the faintest idea of how it can be interacted with or apprehended. Cautioningly, the physicist, G. Bogdarow, says:

One thing we should never forget, in all that follows we have neither a map nor a compass. In our exploration we feel our way about unknowingly in and into the unknown. The physical world has bid us goodbye.

For the physicist, Helmut Satz, this realisation is nothing less than a second Copernican Revolution.

With respect to God and life after death, according to One World Pragmatism, truth is that which is useful and which enables us to survive and this includes the question of whether and in what form Gods exists and whether there is a life after death. As stated at the beginning of this essay, given the state of the environment today, one can conclude that the over simplistic materialist view of the world held by many people today, along with the absurdly primitive idea that the only thing that counts in life is money, is not true for the simple reason that it is causing us to destroy our environmental niche and with it ourselves. One World Pragmatism is thus intended as a pragmatically based alternative in which the non-linear and fragile nature of our environment is implicit. Anthropologically, the importance of a shared set of values for a society is shown by a recent investigation headed by Patrick Savage at Oxford University. Here data drawn from the last 10,000 years was gathered and 414 different societies from 30 different global regions were analysed. The results show that powerful moralising gods and supernatural proto-social mechanisms such as the Buddhist Karma, were almost always a feature of societies in which there were a million or more people. Instead of being driven by fear, as previously thought, the researchers see beliefs in which morals were integral, as being the common bond which enabled different ethnic elements to co-operate, this being the necessary prerequisite for the emergence of a complex society. From the point of view of the societies concerned, the partaking in a shared sense of identity is more important that the degrees of religious conviction held by individuals. As life forms become ever more complex so the nature of problems that require dealing with also become more complex. As individuals and as a society, the point of life is to seek that which is good. Drawing from pragmatism, one can say that the purpose of life for beings such as ourselves, equipped with what scientists call „higher-order consciousness“, is to believe, search and to create. Returning to Gödel, one can see his proof of God as a mathematical equivalent of what the Oxford University investigation arrived at through anthropological comparison. The bottom line is that we must re-discover for ourselves the long forgotten truth that through belief, we can work towards something and setting out on a search, if we are persistent enough and refuse to give up, we can create and find what we are looking for. In this way, through faith and through the above experiments, we can escape the crippling nihilism of modernism and strive for understanding of that which we are a part of and which enables us to be. In the words of Albert Einstein:

Man is a part of the whole that we call „the universe“, a part of space and time enclosed by borders. We experience ourselves, our thoughts and feelings as being separate from all else – a sleigh of hand trick caused by consciousness. This trick is a form of prison known only to ourselves and shared with those to whom we are close to. Our goal must however be to escape from this prison, by furthering the horizons of our empathy so that they include all living things and the whole of nature in its beauty. No one can do this completely but striving for such a state is the beginning of an escape and a basis for inner peace.

Inner peace is a reoccurring theme in the writings of one the most well-known Stoics, the Roman Emperor, Marcus Aurellius. Despite being the ruler of the largest empire on Earth, in his Meditations, or That written unto Himself, Marcus Aurellius was concerned with  modesty, an ethos doing the right thing and the attainment of inner peace. The Stoics believed that everything is rooted in nature and that to live happily, it is essential to understand nature and how the ups and downs of life fit into a wider pattern of which we are but a part. Departing from this realisation, the goal is to minimise negative emotions and maximise positive ones. This is achieved by a continual striving to cultivate a state of eudaimonia, a state in which a good demon holds sway over the human soul as opposed to an evil one and for this, inner peace was seen by Marcus Aurellius as an essential prerequisite. Confronted with challenges, the Stoic endeavors to rise to the occasion and to make the best of any situation. The ultimate ideal is virtue, which can also be described as a nobility of spirit, for with a noble, virtuous spirit that rises to an occasion, the good and happiness will follow. In his Meditations, Marcus Aurellius wrote:

Who are Alexander, Gaius and Pompey when compared with Diogenes, Heraclitus and Socrates? While they (the latter) saw things as they are, their causes and constitutions, the guiding spirits of theit souls were impartial. Were they in any way subject to uncertainty and addiction? (VIII,3)

Worry, fear and the need for material things that have suddenly become unavailable are recurrent themes in the Emperor’s writings and he was eminently aware of the shortness and fragility of life:

The length of a man’s life is on the one hand, only a blip of time, on the other, it is something flowing. Our perception is unclear, our bodily composition entirely corruptible. our soul disorientated, our fate difficult to discern, our voice unclear: put shortly, all bodily things are a river; all mental things are dreams and darkness. Life is war and sojourns of exile, fame is forgetfulness. Is there anything that can help? One thing and one thing alone: philosophy. (II.17)

To this effect he urged:

Think of yourself as one who could die at any time and try to live the rest of your life in tune with nature. (VII.56)

and

Search within. Within is the source of the good, capable of ever flowing, if ever you do search. (VII.59)